
Mishita Dharia - Quadratics
Key Features of Quadratic Relations
The graph of a quadratic relation is called a parabola.
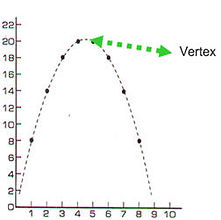
The Vertex
The vertex of a parabola is the maximum (if the graph opens down) or the minimum point (if the graph opens up). It is essentially, the point where the graph changes directions.

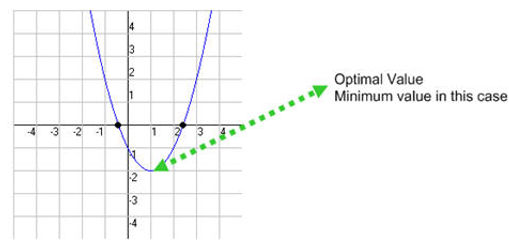
Optimal Value
The optimal value of a quadratic relation is the y-coordinate/k value of the vertex. The optimum value can either be called minimum or maximum. It is called a minimum if the parabola opens up and has a positive a-value and it is called maximum if the parabola opens down and has a negative a-value.
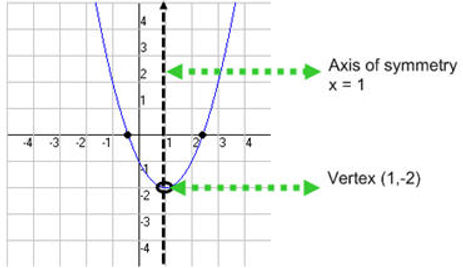
Axis of Symmetry
Every parabola has an axis of symmetry, which is a line the divides the parabola in to perfect halves. The axis of symmetry is the h-value in the vertex, since the vertex of a parabola lies on the axis of symmetry. Therefore the equation of the axis of symmetry is x=h.
X-intercepts
A parabola can have two x-intercepts. These are the points at when the parabola intercepts the x-axis and when y=0.
